Triangles in Trapezoid 1

This is a good starter question. We will see numerous, nice relationships in the trapezoid in the next few problems.

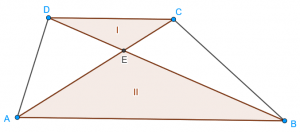
What’s going on: Given a trapezoid with diagonals, prove that the triangles on the bases of the trapezoid are similar.

Student handout: Triangles-in-a-Trapezoid-1

GeoGebra file: here

Teacher Notes

Understanding similarity is very useful (therefore important 😉 ) for solving geometry problems. It could be argued that similarity is more common than congruence.

This is quite easy to prove, using alternate interior angles.

Important/Useful Takeaways for students:

• Always look for similar triangles. They will provide useful relationships.  That is, once we have similar triangles, we can write proportions and these help us.

Significant and interesting results will follow in upcoming problems from this starter similarity relationship.